A triangle is a special right triangle (a right triangle being any triangle that contains a 90 degree angle) that always has degree angles of 30 degrees, 60 degrees, and 90 degrees Because it is a special triangle, it also has side length values which are always in a consistent relationship with one another30 60 90 triangle sides calculator If you just know the length of one "leg" of a 45 45 90 triangle (figure 8), multiply it by the square root of 2 (about 1414) to obtain the hypotenuse length Clicking "RESET" clears all of the boxes Triangles are classified as "special right triangles"Special Triangles Isosceles and Calculator This calculator performs either of 2 items 1) If you are given a right triangle, the calculator will determine the missing 2 sides Enter the side that is known After this, press Solve Triangle 2) In addition, the calculator will allow you to same as Step 1 with a right triangle

30 60 90 Triangle Calculator Formula Rules
30 60 90 triangle side lengths calculator
30 60 90 triangle side lengths calculator-BMI Calculator » Triangle Calculators » Length and Distance Conversions• perimeter = long side short side hypotenuse;
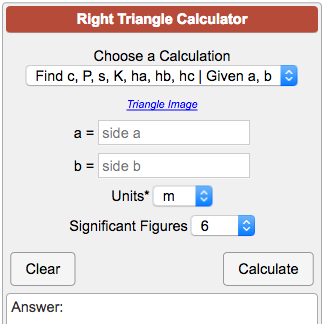



Right Triangles Calculator
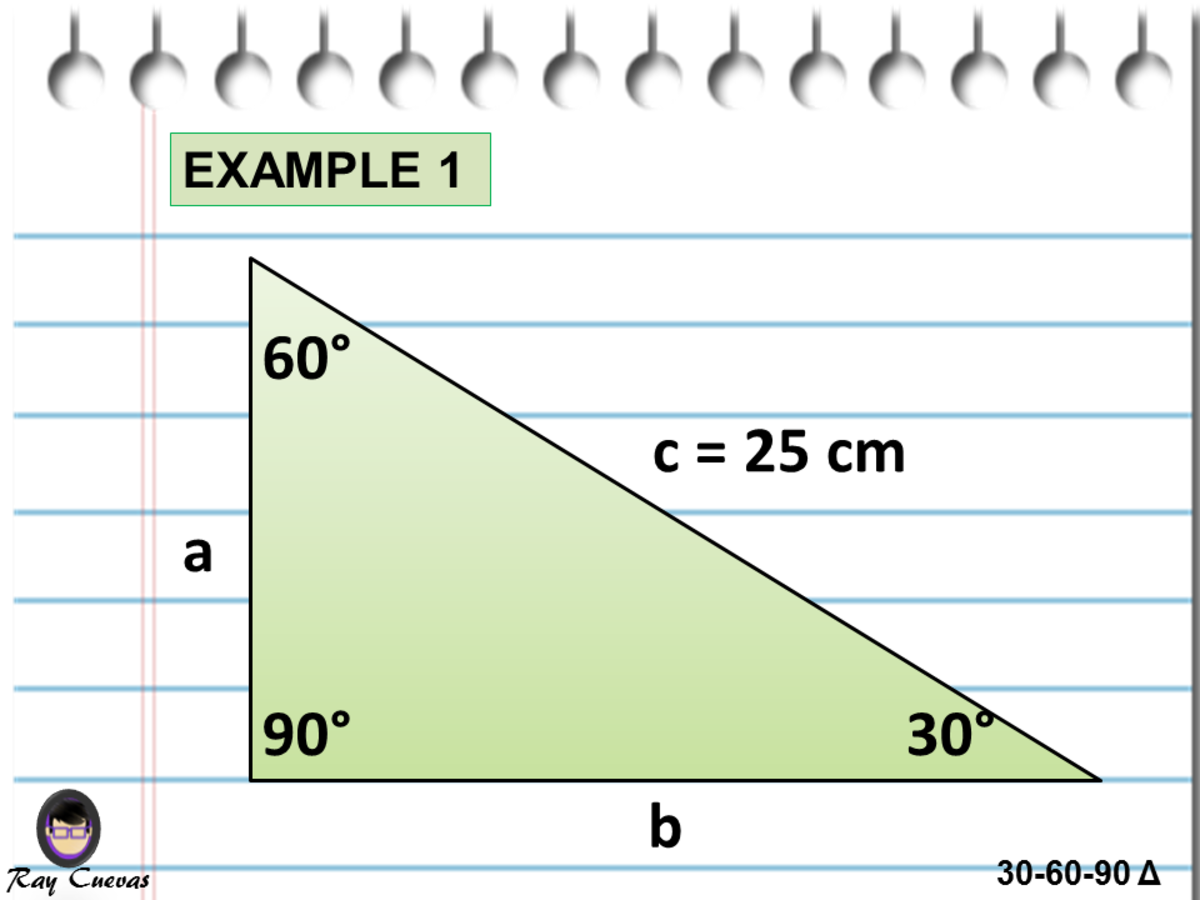
We can use the Pythagorean theorem to show that the ratio of sides work with the basic triangle above a2b2=c2 12(3–√)2=13=4=c2 4–√=2=c Using property 3, we know that all triangles are similar and their sides will be in the same ratioWe know immediately that the triangle is a , since the two identified angles sum to 1° 1 ° 180° − 1° = 60° 180 ° 1 ° = 60 ° The missing angle measures 60° 60 ° It follows that the hypotenuse is 28 m 28 m, and the long leg is 14 m * √3 14 m * 3This calculator exists to compute the area of a triangle, where the three angles of the triangle measure 30, 60, and 90 degrees respectively, and only one other measurement is known It works with a measure of any one side, or by inputting the area or perimeter
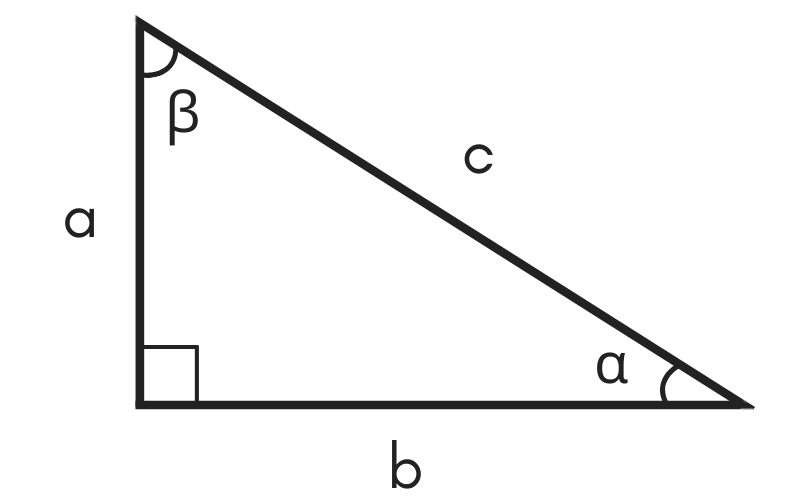
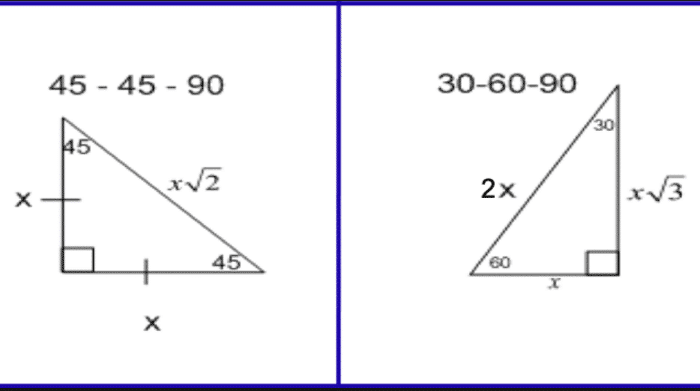
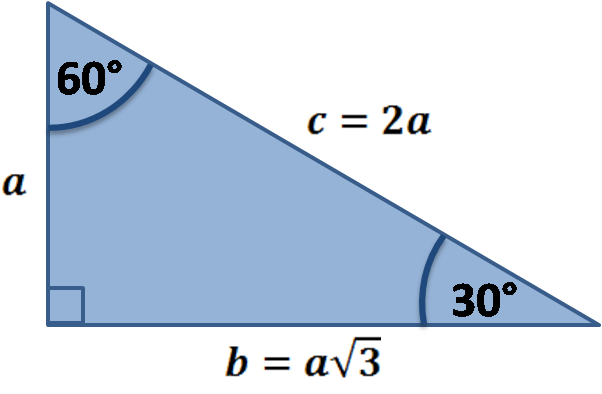
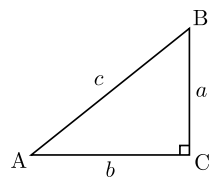
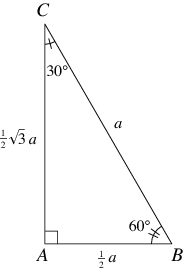
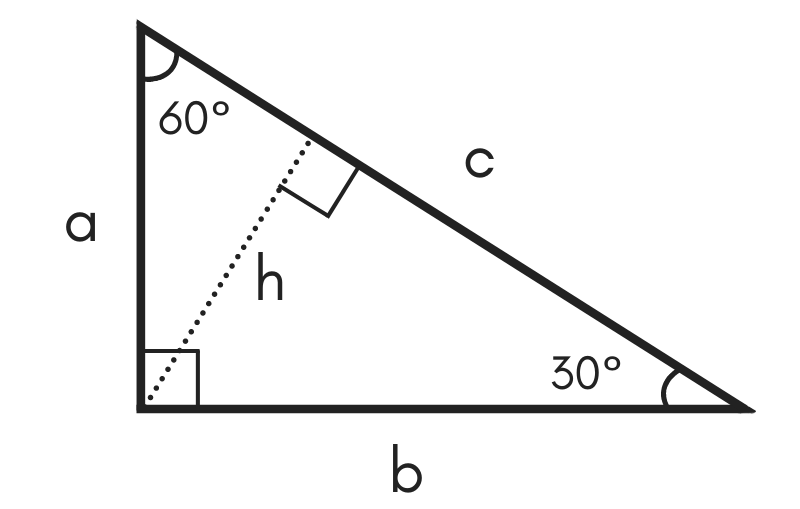
The sides of a right triangle lie in the ratio 1√32 The side lengths and angle measurements of a right triangle Credit Public Domain We can see why these relations should hold by plugging in the above values into the Pythagorean theorem a2 b2 = c2 a2 ( a √3) 2 = (2 a) 2 a2 3 a2 = 4 a2Practice Using the Triangle to Find Side Lengths with practice problems and explanations Get instant feedback, extra help and stepby30° 60° Triangle Calculator Long Side Short Side Hypotenuse Area Cotangent Formulas of triangle with angle 30̊, 60̊ and 90̊ • area = 05 * long side * short side;
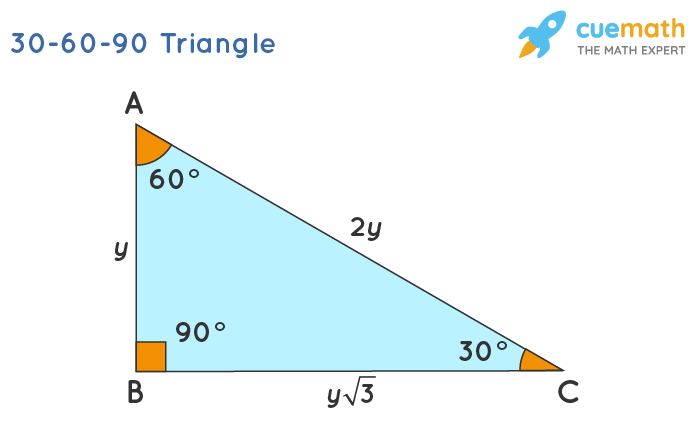
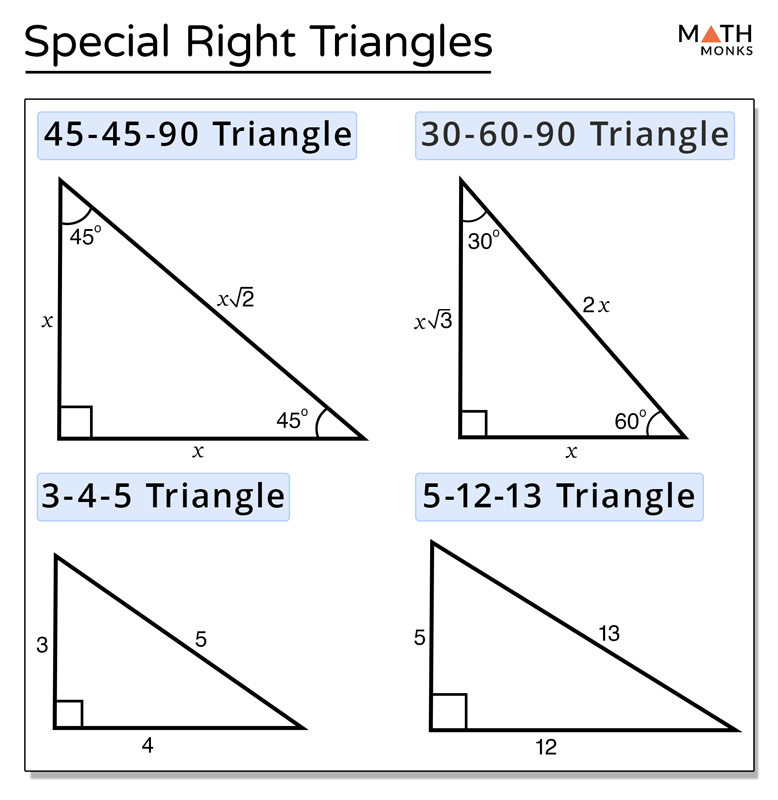
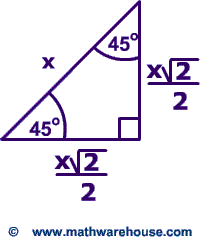
Find the hypotenuse of a triangle with a short side of 3 units Hypotenuse= Step 1 Use the formula 2*s Step 2 2*3 =6 units E2 Find the long side of a thirty sixty ninty triangle with a short side of 3 units Long leg = Step 1 Use the formula short side√ (3 ) Step 2 3√3 units Thanks to this 30 60 90 triangle calculator you find out that shorter leg is 635 in because a = b√3/3 = 11in * √3/3 ~ 635 in hypotenuse is equal to 127 in because c = 2b√3/3 = 2a ~ 127 in area is 349 in² it's the result of multiplyingThe 45°45°90° triangle, also referred to as an isosceles right triangle, since it has two sides of equal lengths, is a right triangle in which the sides corresponding to the angles, 45°45°90°, follow a ratio of 11√ 2 Like the 30°60°90° triangle, knowing one side length allows you to determine the lengths of the other sides



What Actually Is Sin Cos And Tan Can We Calculate It Without A Calculator Quora



Triangle Calculator
The right triangle is a special case triangle, with angles measuring 30, 60, and 90 degrees This free geometry lesson introduces the subject and provides examples for calculating the lengths of sides of a triangle The triangle is a special right triangle, and knowing it can save you a lot of time on standardized tests like the SAT and ACT Because its angles and side ratios are consistent, test makers love to incorporate this triangle into problems, especially on the nocalculator portion of the SATTriangle in trigonometry In the study of trigonometry, the triangle is considered a special triangleKnowing the ratio of the sides of a triangle allows us to find the exact values of the three trigonometric functions sine, cosine, and tangent for the angles 30° and 60° For example, sin(30°), read as the sine of 30 degrees, is the ratio of the side



30 60 90 Special Right Triangle Calculator Inch Calculator



30 60 90 Triangle Definition Theorem Formula Examples
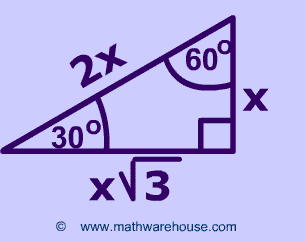
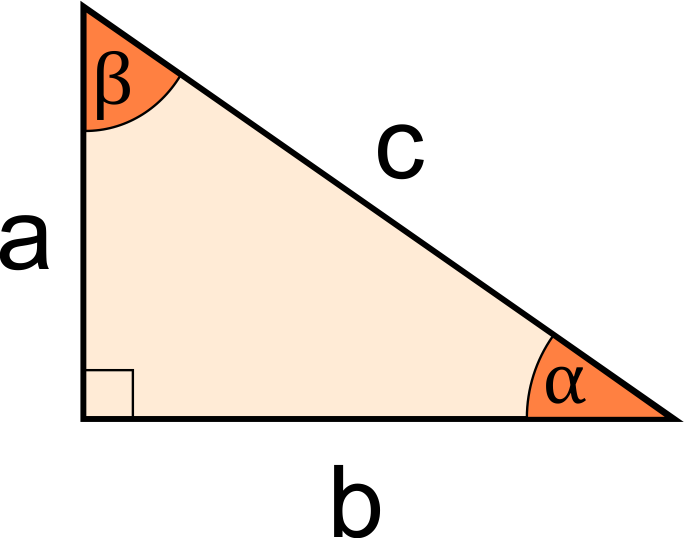
This calculator calculates for the length of one side of a right triangle given the length of the other two sides A right triangle has two sides perpendicular to each other Sides "a" and "b" are the perpendicular sides and side "c" is the hypothenuse Enter the length of any two sides and leave the side to be calculated blankEnter 1 out of 3 to solve for the other 2 missing sides Special right triangle 30 60 90 is one of the most popular right triangles The 30 60 90 right triangle is a special case triangle with angles measuring 30 60 and 90 degrees If you want to read more about that special shape check our calculator dedicated to the 30 60 90 triangleThe graphics posted above show the 3 cases of a 30 60 90 triangle If you know just 1 side of the triangle, the other 2 sides can be easily calculated For example, if you only know the short side (figure5), the medium side is found by multiplying this by the square root of 3 (about 1732) and the hypotenuse is calculated by multiplying the short side by 2




45 45 90 Triangle Calculator Formula Rules




Right Triangles Calculator
Trigonometry Examples Popular Problems Trigonometry Solve the Triangle tri {} {30} {} {60} {} {90} Side Angle b = c = a = A = 30 B = 60 C = 90 Side Angle b = c = a = A = 30 B = 60 C = 90 There is not enough information to solve for all of the sides and angles of this triangle Unknown triangleSo draw a perpendicular to the base, which also bisects both the third side as well as the 1° vertex angle like this It bisects the 1° into two 60° angles like this Let each of the two halves of the third side be x Now for the right triangle on the left Since this is a 30°60°90° right triangle, we know that the shorter leg (the 45 45 90 triangle calculator is a dedicated tool to solve this special right triangle Find out what are the sides, hypotenuse, area and perimeter of your shape and learn about 45 45 90 triangle formula, ratio and rules If you want to know more about another popular right triangles, check out this 30 60 90 triangle tool and the calculator for
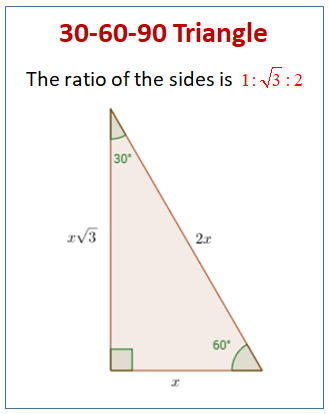



30 60 90 Special Right Triangles Videos Worksheets Examples Solutions




30 60 90 Triangle Sides Examples Angles Full Lesson
Dimensions of 30 60 90 triangle What is 30 60 90 triangle What are the formulas for a 30 60 90 triangle Hi Ron, it depends on which side is the base If the opposite side at the angle of 30 degrees in a triâgle has a length unit 1, the hypotenuse has the duration 2 units and the third side has length unitsFind the length of the side x Solution 1 Since the triangle is equilateral, it is also equiangular, and therefore the the angle at B is 60° The height of a triangle is the straight line drawn from the vertex at right angles to the base Therefore, triangle ADB is a triangleCalculator Use An equilateral triangle is a special case of a triangle where all 3 sides have equal length and all 3 angles are equal to 60 degrees The altitude shown h is h b or, the altitude of b For equilateral triangles h = ha = hb = hc If you have any 1 known you can find the other 4 unknowns So if you know the length of a side = a




The Complete Guide To The 30 60 90 Triangle




A Quick Guide To The 30 60 90 Degree Triangle Dummies
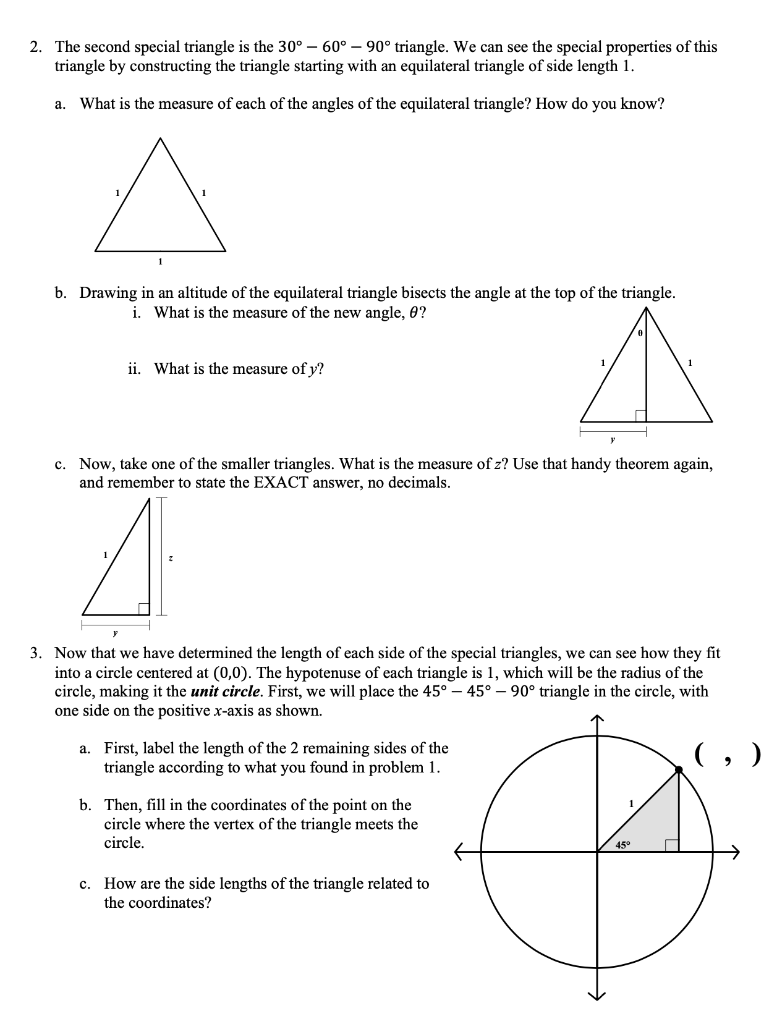
Special Triangles Isosceles and Calculator This calculator performs either of 2 items 1) If you are given a right triangle, the calculator will determine the missing 2 sides Enter the side that is known After this, press Solve Triangle 2) In addition, the calculator will allow you to same as Step 1 with a right triangleCalc third side of scalene forming lift arm of aircraft dolly/jack (from two known sides and included angle) To improve this 'Scalene triangle Calculator', please fill in questionnaire Age Under years old years old level 30 years old level 40 years old level 50 years old level 60 years old level or over OccupationA triangle is a special triangle since the length of its sides is always in a consistent relationship with one another In the belowgiven triangle ABC, ∠ C = 30°, ∠ A = 60°, and ∠ B = 90° We can understand the relationship between each of the sides



30 60 90 And 45 45 90 Triangle Calculator



Triangle Calculator
Inch Angle Calculator Isosceles Triangles Measure Angles and Side Lengths by entering 2 known values Enter Side Lengths and either top Angle or Base length to calculate all other side lengths, angles, triangle height and area Top Angle Base Angles 30° Height 3' Area 1559 ft² Trigonometry angle calculator diagram



The Easy Guide To The 30 60 90 Triangle




30 60 90 Triangle Theorem Ratio Formula Video
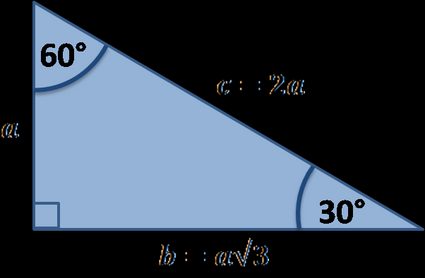



30 60 90 Triangle Calculator Formula Rules




Right Angle Triangle Trigonometry Review Ck 12 Foundation
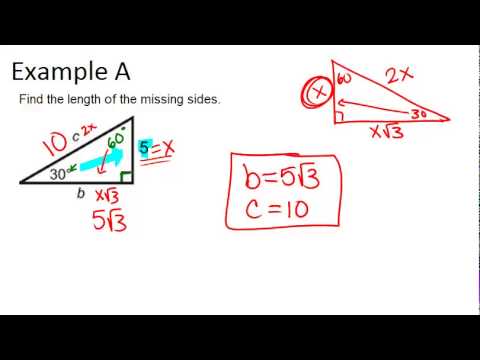



30 60 90 Right Triangles Examples Geometry Concepts Youtube



30 60 90 Special Right Triangle Calculator Inch Calculator



Right Triangles Gmat Free




30 60 90 Triangle Theorem Properties Formula Video Lesson Transcript Study Com



Special Right Triangles Definition Formula Examples



The Length Of The Hypotenuse Of A 30 60 90 Triangle Is 12cm What Is The Length Of The Longer Leg What Is The Length Of The Shorter Leg Quora



30 60 90 And 45 45 90 Triangle Calculator



A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation




30 60 90 Triangle Theorem Properties Formula Video Lesson Transcript Study Com




Right Triangle Calculator Find A B C And Angle



1




Special Right Triangles Calculator Formula Rules



The Easy Guide To The 30 60 90 Triangle




30 60 90 Triangle Theorem Ratio Formula Video
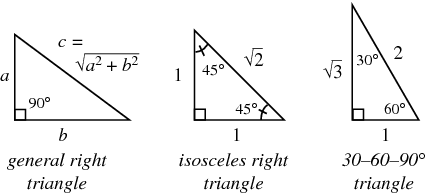



Right Triangle From Wolfram Mathworld



30 60 90 And 45 45 90 Triangle Calculator




30 60 90 Triangle Calculator Formula Rules




Special Right Triangles In Geometry 45 45 90 And 30 60 90 Youtube



What Are The Properties Of A 30 60 90 Triangle Quora




A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation




View Question Practice Problems 1 In A 30 60 90 Triangle What Is The Length Of The Hypotenuse When The Shorter Leg Is 8m




A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation




30 60 90 Triangle Calculator Formula Rules




Velocity
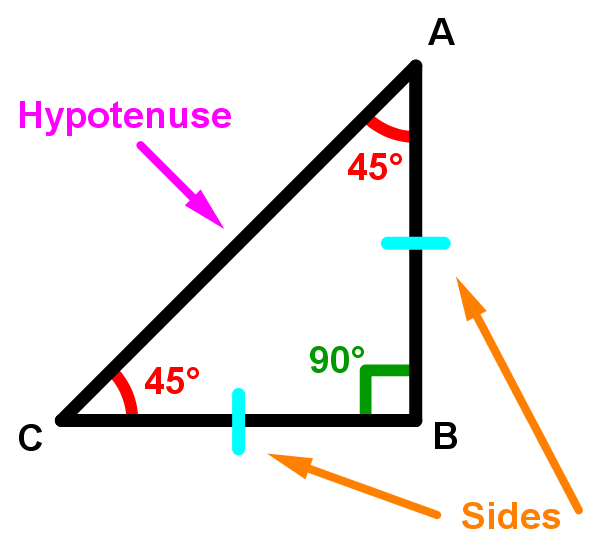



How To Use The Special Right Triangle 45 45 90 Studypug
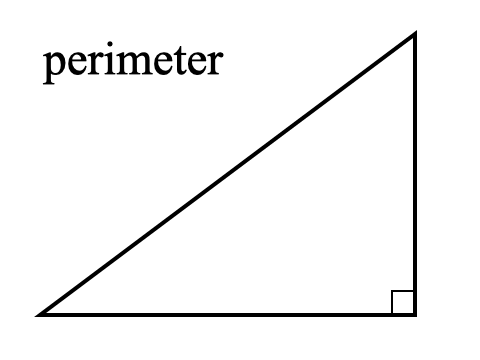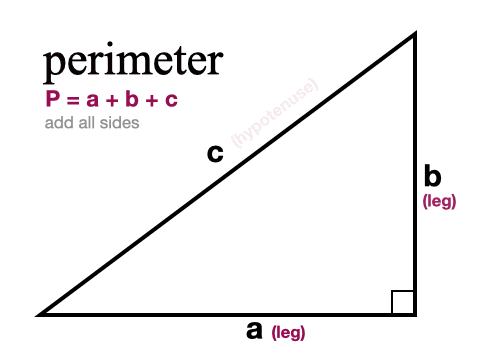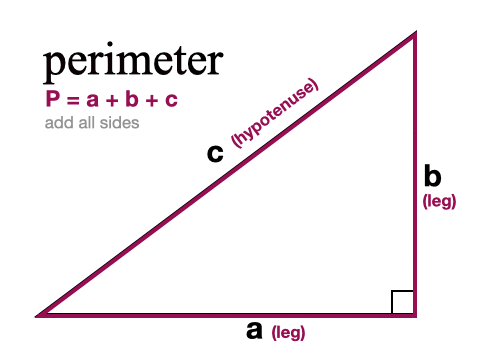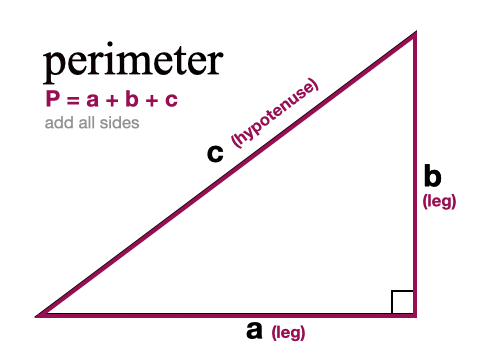



Right Triangle Calculator Pi Day




Special Right Triangles Review Article Khan Academy




30 60 90 Triangle Calculator Purecalculators



1




Special Right Triangles Fully Explained W 19 Examples




30 60 90 Triangles Special Right Triangle Trigonometry Youtube




How To Calculate The Missing Side Length Of A Triangle Youtube



Special Triangles Isosceles And 30 60 90 Calculator



Special Right Triangles Complete Reference Guide The Education



30 60 90 Triangle Calculator Online Discount Shop For Electronics Apparel Toys Books Games Computers Shoes Jewelry Watches Baby Products Sports Outdoors Office Products Bed Bath Furniture Tools Hardware



30 60 90 Right Triangle Calculator



30 60 90 Triangle Unit Circle



30 60 90 Triangle From Wolfram Mathworld




A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation




A Quick Guide To The 30 60 90 Degree Triangle Dummies




How To Work With 30 60 90 And 45 45 90 Degree Triangles Dummies
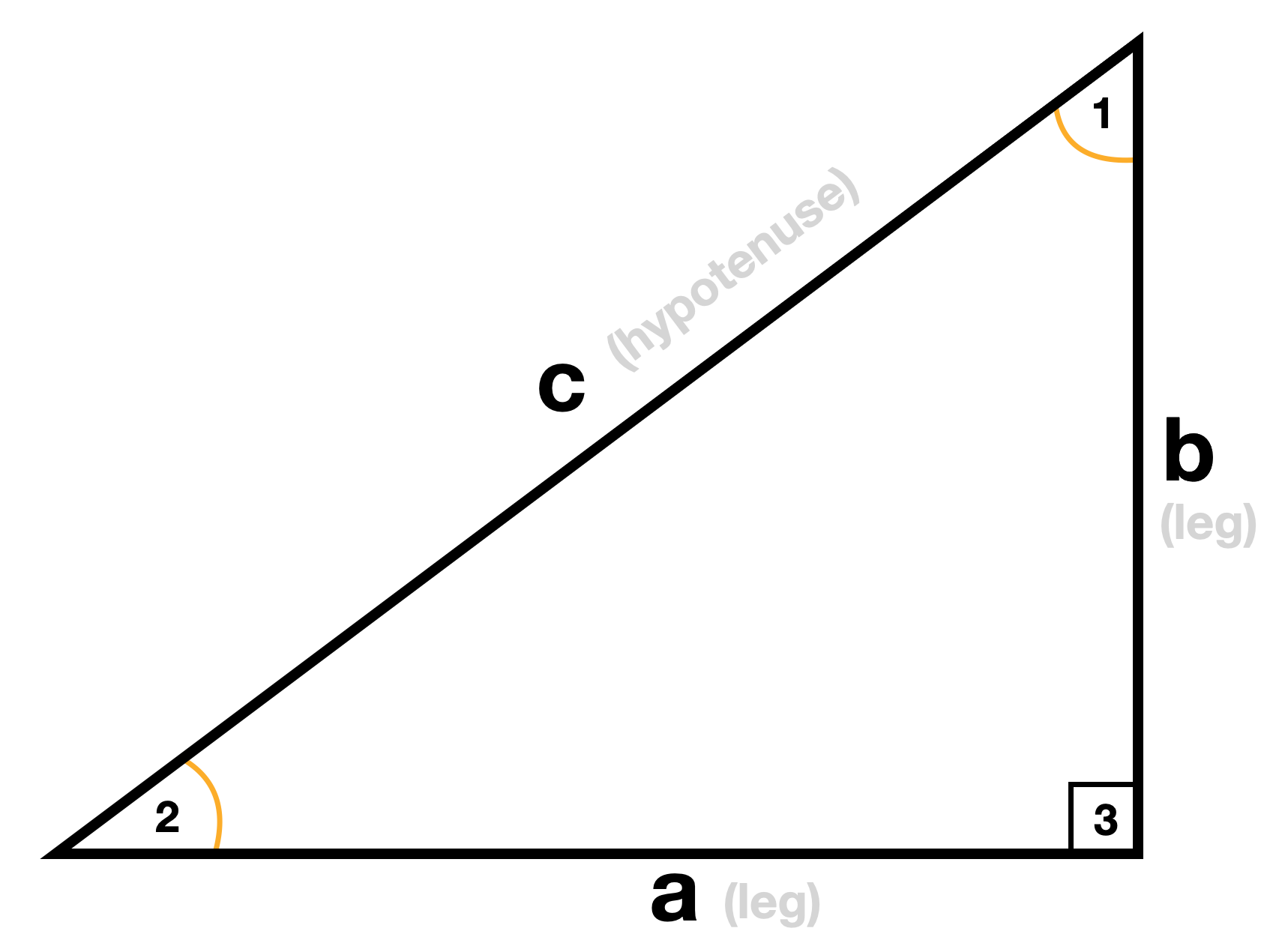



Right Triangle Calculator Pi Day



Special Right Triangles Formulas 30 60 90 And 45 45 90 Special Right Triangles Examples Pictures And Practice Problems



4 Special Triangles And The Unit Circle No Chegg Com




Triangle Calculator Square Footage Area



45 45 90 And 30 60 90 Triangles Zona Land Education




30 60 90 Triangle Theorem Ratio Formula Video




The Complete Guide To The 30 60 90 Triangle




30 60 90 Special Right Triangle Calculator General Knowledge Book Right Triangle Electrical Engineering Books



30 60 90 Special Right Triangle Calculator Inch Calculator



Special Right Triangles Formulas 30 60 90 And 45 45 90 Special Right Triangles Examples Pictures And Practice Problems



30 60 90 Triangle Rules




30 60 90 Triangle Calculator Formula Rules




30 60 90 Triangle Explanation Examples



Right Triangle Calculator Find A B C And Angle



Find Sides Of 45 45 90 30 60 90 Right Triangles Youtube



Triangle 30 60 Calculator Endmemo



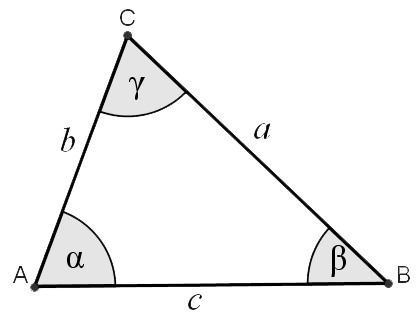
Right Triangle Calculator Solve Any Edge Or Angle Inch Calculator



30 60 90 Triangle Theorem Properties Formula Video Lesson Transcript Study Com



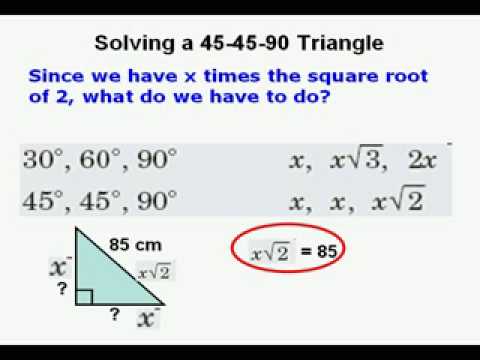
30 60 90 Triangle Example Problem Video Khan Academy



The Easy Guide To The 30 60 90 Triangle




30 60 90 Right Triangles Solutions Examples Videos



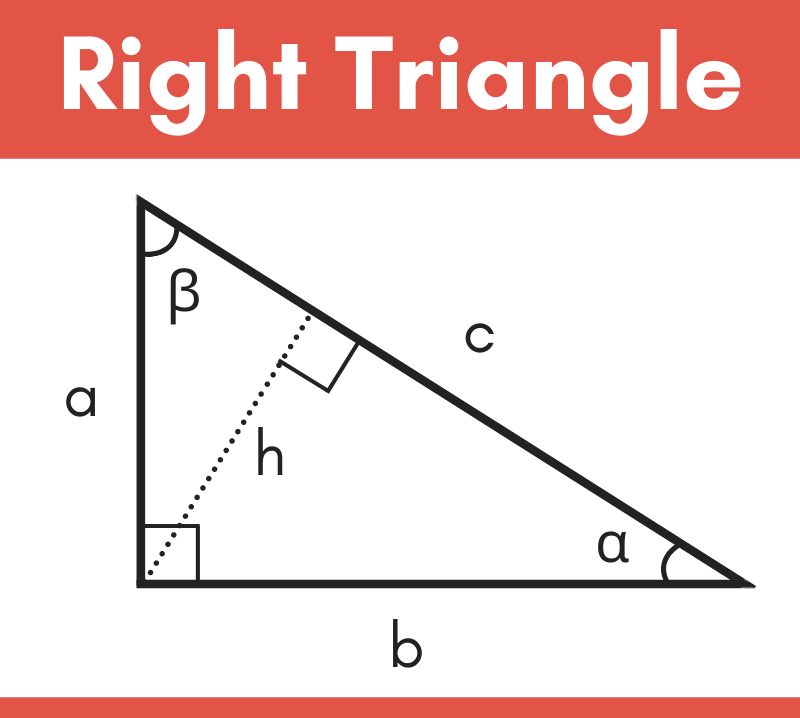
Triangle Calculator



Special Right Triangle 30 60 45 45 37 53 Elearning




30 60 90 Triangle Calculator Formula Rules




How To Work With 30 60 90 Degree Triangles Education Is Around




Special Right Triangles 30 60 90 And 45 45 90 Triangles Youtube



Solve A 30 60 90 Triangle With Gradea
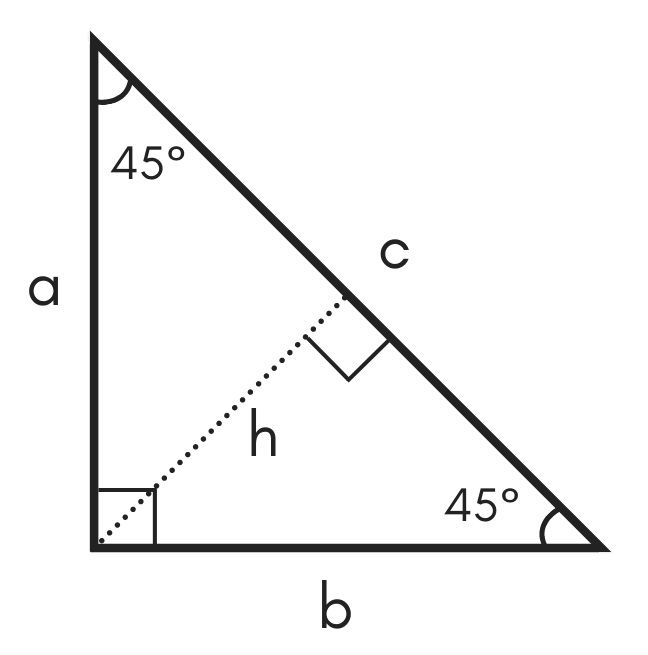



45 45 90 Special Right Triangle Calculator Inch Calculator




30 60 90 Right Triangle Side Ratios Expii
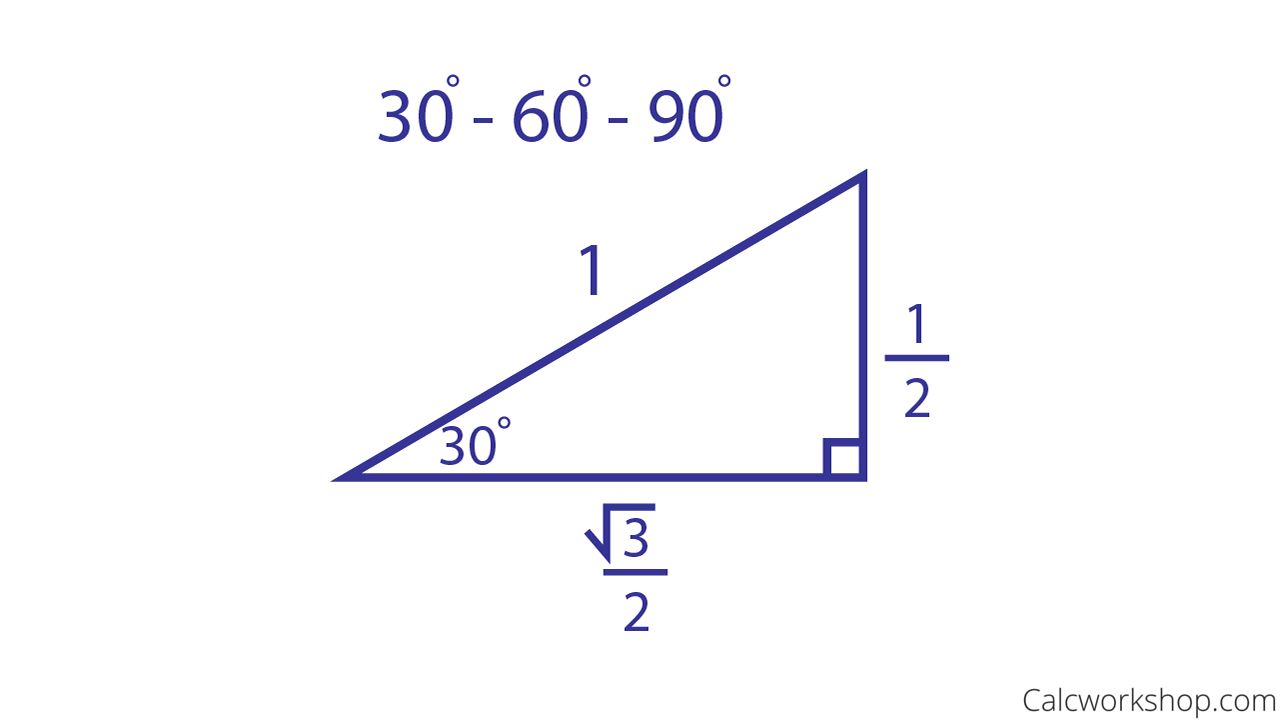



Unit Circle W Everything Charts Worksheets 35 Examples




Activity 22 Construct A 30 60 90 Triangle Geogebra



3




30 60 90 Right Triangles Free Math Help
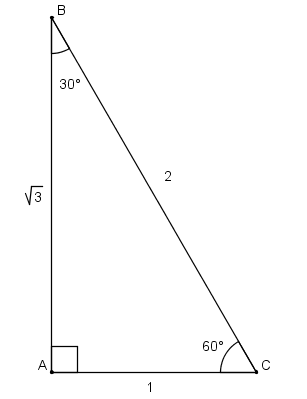



Find The Value Of The Sine Or Cosine Functions Of An Angle Given A Point On Its Terminal Side Precalculus



30 60 90 Triangle Rules Calculator Formula The Free Calculator
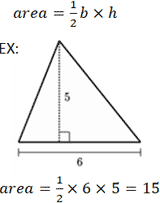



Triangle Calculator




30 60 90 Triangle Theorem Ratio Formula Video




Special Right Triangles Fully Explained W 19 Examples
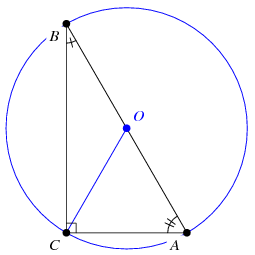



30 60 90 Triangle From Wolfram Mathworld




30 60 90 Triangle Theorem Ratio Formula Video



30 60 90 And 45 45 90 Triangle Calculator
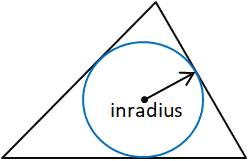



Triangle Calculator



13 3 2 The 30 60 90 Triangle Graphing Calculator By Mathlab User Manual



13 3 2 The 30 60 90 Triangle Graphing Calculator By Mathlab User Manual




30 60 90 Special Right Triangle Calculator Inch Calculator
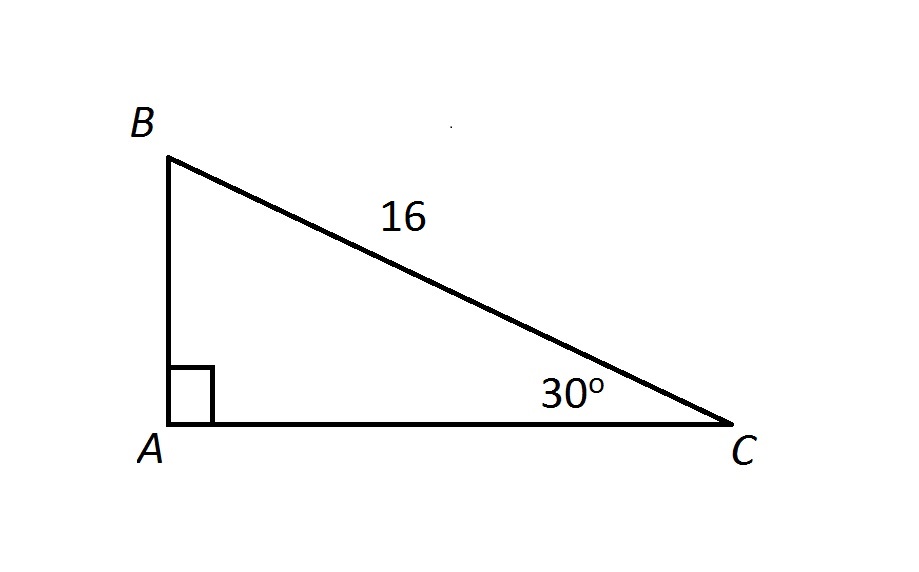



Special Triangles Hiset Math
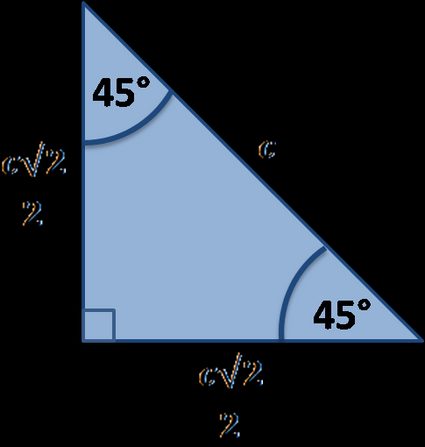



45 45 90 Triangle Calculator Formula Rules


